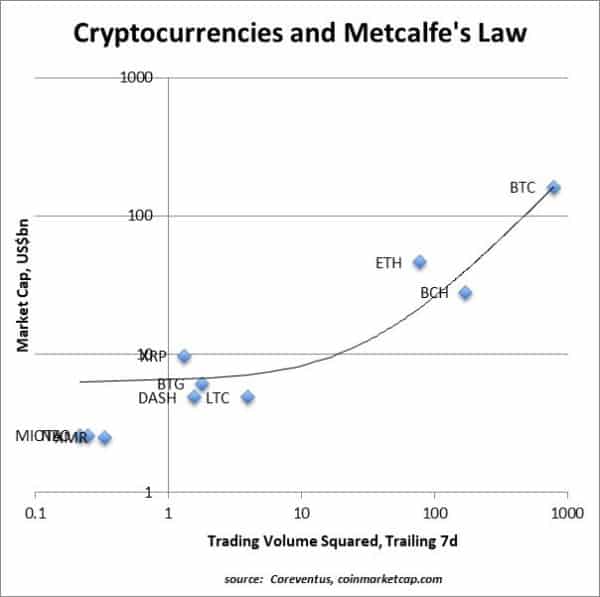
Valuing Cryptocurrencies Using Metcalfe’s Law

One way to value cryptocurrencies is by Metcalfe’s Law, which states that the value of a network is proportionate to the square of the number of users of the network.

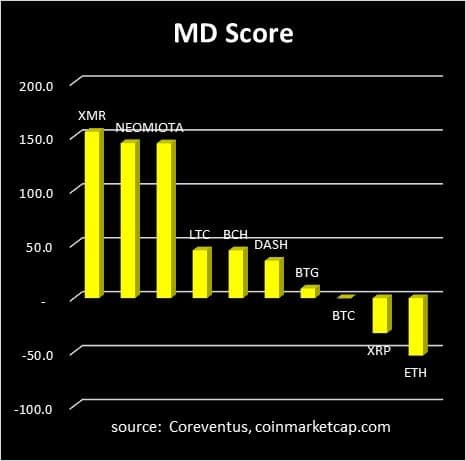
We use this concept to value cryptocurrencies relative to each other. Using the MD Score, derived from the relative valuation based on trading volume and market cap, Monero has the most upside, Bitcoin Cash the most upside of the larger currencies, and Ethereum the most downside.

METCALFE’S LAW AS A VALUATION METRIC

To analyze cryptocurrencies as a network, we use recent trading volumes -here we use trailing seven days- as a proxy for users on network. We then square this, as per Metcalfe’s Law, and compare this to the market cap of the coin – the results are on the chart above.

Cryptocurrencies above the regression line are overvalued (by this analysis), and those below the line are undervalued.

THE MD SCORE: HOW TO RANK CRYPTOCURRENCIES

The MD Score is a way of ranking cryptocurrencies using Metcalfe’s Law. It’s quite simple -the score measures the distance, up or down, between what the currency’s market cap and the market cap as calculated by the regression equation and based on the trading volumes.

The higher the MD Score, the more upside potential the currency has.

Mean Reversion is the governing philosophy here. Yes, it doesn’t always hold -Amazon (AMZN – $1186) just seems to keep going up for example. However, it is a powerful force in financial markets.

As we can see by the above chart, some of the smaller currencies show considerable upside potential, particularly IOTA (MIOTA), Neo (NEO) and Monero (XMR).

Of the larger currencies, Bitcoin Cash (BCH) has the most upside potential. Ethereum (ETH) has the most downside.

Currencies (Ranked by Market Cap)

BTC Bitcoin
ETH Ethereum
BCH Bitcoin Cash
MRipple Ripple
BTG Bitcoin Gold
LTC Litecoin
DASH Dash
NEO * NEO
XMR Monero
MIOTA IOTA

source: coinmarketcap.com
Metcalfe’s Law

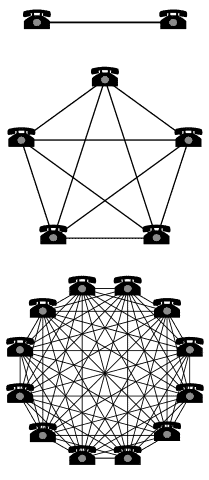
Robert Metcalfe, a co-inventor of the Ethernet Protocol and an early developer of the Internet and its predecessor, ARPAnet, posited that the value of a network was proportionate to the square of the number of users or devices on that network.

The Law makes intuitive sense – connections grow much faster than devices as the network expands.